MATERIALS SCIENCE AND CHEMISTRY
Quantum Monte-Carlo and Exact Diagonalization Studies of Correlated Electron Systems
Principal Investigator:
Prof. Dr. F. F. Assaad and Prof. Dr. W. Hanke
Affiliation:
Institut für Theoretische Physik und Astrophysik, Universität Würzburg (Germany)
Local Project ID:
h014z
HPC Platform used:
SuperMUC of LRZ
Date published:
Correlated electron systems are defined by the notion that the whole is more than the sum of its parts. One can mention collective excitations in continuous symmetry broken phases such as magnetism or superconductivity, exotic phenomena such as the fractionalization of the electron with elementary charge e and spin ℏ/2 into a spinon, carrying spin only, and a holon, carrying charge only, or states that can only be characterized by global topological quantities.
The cornerstone to realize these states of matter is the correlation between electrons mediated by the Coulomb interaction, or, coupling to the lattice in terms of the electron-phonon interaction. Emergent collective phenomena is, strictly speaking, only well defined in the thermodynamic limit. This confronts us with a numerical challenge: taking into account correlation effects between an infinite number of electrons. It is clearly impossible to reach the thermodynamic limit, both experimentally and numerically, but large enough systems will exhibit collective phenomena above an energy scale inversely proportional to the volume of the system. Numerically, this amounts to the importance of being able to carry out simulations on large systems, which implies the ability to harness the power of supercomputing architectures.
On classical computers the computational effort required to carry out simulations of many-body quantum mechanical systems generically scales exponentially with the number of particles. This is certainly the case when exact diagonalization methods are used put to use. There is however an ever growing number of non-trivial model Hamiltonians for which stochastic quantum Monte Carlo algorithms with polynomial scaling in the particle number can be formulated. It is in this domain where we have achieved most of our progress, both at the algorithmic level and in our physical understanding.
Model calculations are particularly relevant for our understanding of low energy phenomena. They are essential for computing critical phenomena and associated critical exponents, which define universality classes. As their name suggests, critical exponents do not depend on the short distance details of the model under consideration. In this domain, and with the help of supercomputing resources of the LRZ, we have greatly progressed. As a concrete example, one can mention numerical simulations of Graphene systems as modeled by a tight binding Hamiltonian on the Honeycomb lattice supplemented by the Coulomb repulsion. This model is relevant both for solid state and high energy physics since in the absence of interactions the low energy effective action is that of relativistic electrons as described by the Dirac equation.
One of the key questions is to understand the mechanism of mass generation in the presence of the Coulomb repulsion. In a number of papers [1,2,3] we have pinned down the universality class of this transition and estimated the critical exponents. In the future, more work at the algorithmic level will be required so as to achieve larger lattices such as to pin down more precisely the value of the critical exponents.
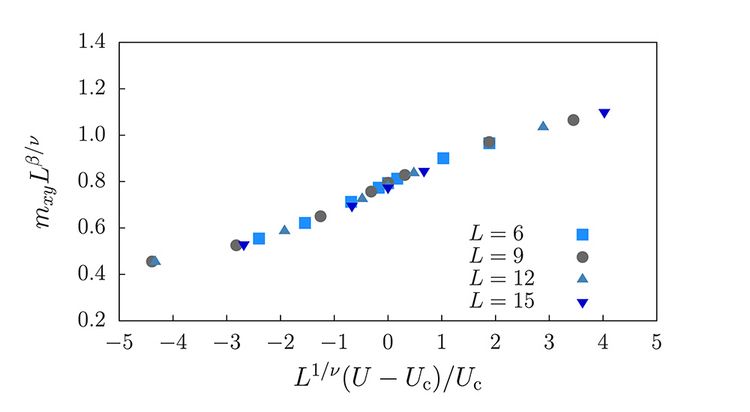
Figure 01: Simulations of the graphene tight binding Hamiltonian on the Honeycomb lattice with a Coulomb repulsion. Using exponents of the first order epsilon expansion we can obtain a data collapse. This data has been published in [1]. Further work aimed at pinning down the critical exponents for the so called Hubbard model on the Honeycomb lattice which is believed to be in the same universality class can be found in [2,3].
Copyright: Institut für Theoretische Physik und Astrophysik, Universität WürzburgReferences:
[1] M. Hohenadler, F. Parisen Toldin, I. F. Herbut, and F. F. Assaad
Phase diagram of the Kane-Mele-Coulomb model,
Phys. Rev. B 90 (2014), 085146. (Editor’s Suggestion)
[2] Fakher F. Assaad and Igor F. Herbut,
Pinning the order: The nature of quantum criticality in the hubbard model on honeycomb lattice,
Phys. Rev. X 3 (2013), 031010.
[3] Francesco Parisen Toldin, Martin Hohenadler, Fakher F. Assaad, and Igor F. Herbut,
Fermionic quantum criticality in honeycomb and π-flux Hubbard models: Finite-size scaling of renormalization group-invariant observables from Quantum Monte Carlo,
Phys. Rev. B 91 (2015), 165108.
Scientific Contact:
Florian Goth
Institut für Theoretische Physik und Astrophysik, Universität Würzburg
Am Hubland, D-97074 Würzburg/Germany
e-mail: Florian.Goth [@] physik.uni-wuerzburg.de
January 2016