MATERIALS SCIENCE AND CHEMISTRY
Frequency-Dependent Dielectric Polarizability of Nanocolloids and Polyelectrolytes in Electric Fields
Principal Investigator:
Jiajia Zhou, Friederike Schmid
Affiliation:
Institute of Physics, Johannes Gutenberg University Mainz (Germany)
Local Project ID:
CCAC
HPC Platform used:
Hazel Hen of HLRS
Date published:
Being able to handle and manipulate large molecules or other nano-objects in a controlled manner is a central ingredient in many bio- and nanotechnological applications. One increasingly popular approach, e.g., in microfluidic setups, is to use dielectrophoresis. Here, the nano-objects are exposed to an alternating electric field, which polarizes them. Depending on the polarization, they can then be grabbed and moved around or trapped by an additional field. However, the mechanisms governing the polarization of the objects, which are typically immersed in a salt solution, are very complicated. Simulations allow to disentangle the different processes that contribute to the polarizability and to assess the influence of key factors such as AC frequency, salt concentration, or salt diffusivity.
The polarization of nano-objects in salt solution (electrolytes) in alternating electric fields (AC fields) is the result of a complex interplay of competing processes, involving, among other, the motion of ions in free solution, the motion of ions along the surface of the nano-objects, the motion of nano-objects, the deformation of nano-objects. All these processes have different speed, i.e., different characteristic time scales. At very high AC frequencies, they are all suppressed and the polarizability drops to zero. However, at intermediate frequencies, they contribute with different weights. From an application point of view, this opens the interesting perspective that the AC frequency can be used to manipulate and tune the local charge structure in the vicinity of the nano-object and the resulting polarization.
The goal of the project was to disentangle and analyze the key processes contributing to the polarizability of nano-objects, in order to obtain basic insights into the dynamics of complex charged fluids and to provide guidance in the design of future manipulation strategies. Two types of nano-objects were studied: Nanocolloids and charged polymers (polyelectrolytes). Even though coarse-grained models were used, the study required extensive simulations and would not have been possible without the use of supercomputer resources.

Fig. 1: Snapshot of a Nanocolloid (left) and polyelectrolyte (right) in electrolyte solution. Only nano-objects and ions are shown, in addition, the system is filled with fluid particles. © image left: JGU, Mainz © image right: Reproduced with permission from J. Electrochem. Soc., 166, B3194 (2019). Copyright 2019, The Electrochemical Society.
The simulations were based on ‘’Dissipative particle dynamics’’ (DPD), and the fluid particles are represented by simple ‘’DPD’’-beads which interact with each other only by friction. Such models have proven to be efficient for simulating fluid flow phenomena on nanometer scales or higher. Additionally, a method was developed which allows avoiding the use of overly bulky explicit salt ions [10]. The use of this method turned out to be crucial for the success of the polyelectrolyte studies [1].
Put simply, the processes determining the polarizability of nano-objects – as observed in the simulations - can be summarized as follows: First, if electric fields are applied, both charged nano-objects and the ions in solution are set into motion, in opposite directions depending on their charge. Nanocolloids are then obstacles for the salt ions. As a result they are polarized even if they are not charged! This is because the ions accumulate at the front and back side (Fig. 2, top).
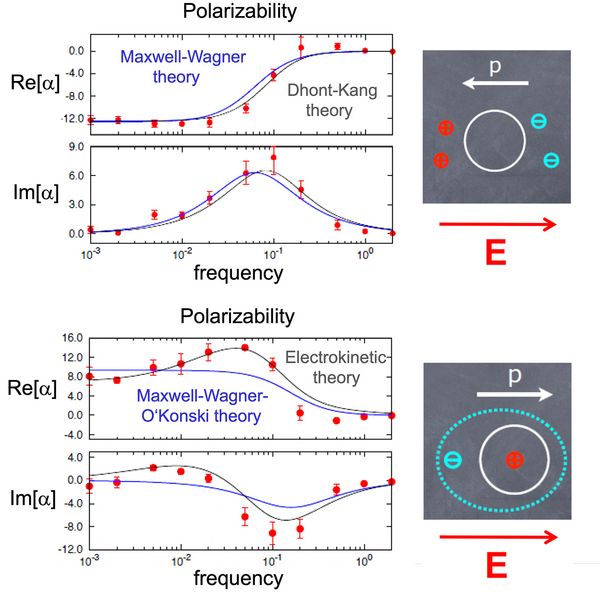
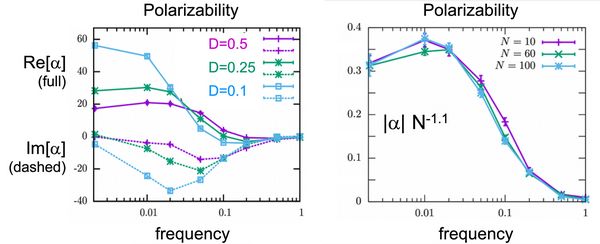
Fig. 2: Top: Frequency-dependent dielectric polarizability of the uncharged nanocolloid [7]. Even an uncharged colloid gets polarized in an external electric field. This is because the colloid is an obstacle for the ions in solution, therefore they accumulate in front of it. The polarization is opposite to the applied field. At high AC frequencies, the ions do not have enough time to follow the field, and the polarization vanishes. Bottom: Frequency-dependent dielectric polarizability of the charged nanocolloid [6]. Here, the electric field polarizes the nanocolloid and the surrounding counterion layer. The polarization is in the direction of the field. At high AC frequencies the polarization vanishes as in Fig. 2. At low AC frequencies, it is also reduced, because it is counterbalanced by salt ion diffusion . (Theory: Dhont & Kang, Eur. Phys. J. E 2010)
Copyright: Johannes Gutenberg University, MainzSecond, if the colloids are charged, another mechanism comes into play and dominates: Charged particles are surrounded by a layer of oppositely charged ions (the electric double layer, EDL). This layer gets distorted in the presence of electric fields. The resulting polarization is opposite to that found for uncharged colloids (Fig. 2, bottom).
Third, ions are also driven from regions with high salt concentrations (thick EDL) to regions with low salt concentrations (thin EDL), which counteracts the distortion of the EDL. However, this can only happen if the ions have time to diffuse along the side of the colloid, i.e., at low frequencies. Therefore, the polarizability initially increases with the frequency until, at very high frequencies, it vanishes again because the ions don’t have time to follow the electric field at all (Fig. 2, bottom).
In the case of colloids, analytical theories can be found in the literature which can be tested against the simulations. In particular, the electrokinetic theory accounts for all mechanisms of polarization described above and shows very good agreement with the simulation results (grey lines in Figs. 2 and 3). For flexible polyelectrolytes, the situation is much more complicated and developing analytical theories is more difficult. Nevertheless, the simulation results show that the behavior of polarizability as a function of the frequency is very similar to that observed for colloids, suggesting that the main mechanisms of polarization are the same (Fig.3). Moreover, the simulations show that the polarizability scales in a slightly superlinear manner with the size of the molecule in agreement with theoretical expectations for short chains. Theoretically, one expects linear scaling in the limit of very long chains.
Thus the simulations have helped to clarify the polarization mechanisms of charged nano-objects in electrolyte solutions and validated existing theories for colloidal dispersions. Even though these theories were originally developed for micrometer-size colloids, the simulations show that they can also be used to describe nano-objects such as, e.g., compact proteins or protein complexes. The polyelectrolyte studies showed that the basic mechanisms are similar for rigid and deformable nano-objects. Nevertheless, many interesting open questions remain here, e.g., regarding the role of solvent quality [1] and chain stiffness, which will require further studies.
References: Results obtained by simulations at HLRS
- G. Jung, S. Kasper, F. Schmid, “Frequency-dependent dielectric polarizability of flexible polyelectrolytes in electrolyte solution: A Dissipative Particle Dynamics simulation”, J. Electrochem. Soc. 166, B3194-B3202 (2019).
- J. Zhou, F. Schmid, “A New Colloid Model for Dissipative-Particle-Dynamics Simulations”, in “High Performance Computing in Science and Engineering ’15”, pp. 89-99, Nagel, Wolfgang E., Kröner, Dietmar H., Resch, Michael M. (Eds.) (Springer, 2015).
- J. Zhou and F. Schmid, “Computer simulations of single particles in external electric fields”, Soft Matter 11, 6728 (2015).
- J. Zhou, J. Smiatek, E.S. Asmolov, O.I. Vinogradova, F. Schmid, “Application of Tunable-Slip Boundary Conditions in Particle-Based Simulations”, in “High Performance Computing in Science and Engineering ’14”, pp. 19-30, Nagel, Wolfgang E., Kröner, Dietmar H., Resch, Michael M. (Eds.) (Springer, 2015).
- J. Zhou, F. Schmid, Computer simulations of charged colloids in alternating electric fields, Eur. Phys. J.: Special topics 222, 2911 (2013).
- J. Zhou, R. Schmitz, B. Dünweg, F. Schmid, “Dynamic and dielectric response of charged colloids in electrolyte solutions to external electric fields”, J. Chem. Phys. 139, 024901 (2013).
- J. Zhou, F. Schmid, “AC-field induced polarization for uncharged colloids in salt solution: A Dissipative Particle Dynamics simulation”, Eur. Phys. J. E 36, 33 (2013).
- J. Zhou, F. Schmid, “A Dissipative-Particle-Dynamics Model for Simulating Dynamics of Charged Colloids”, in “High Performance Computing in Science and Engineering ’13”, pp. 5-18, Nagel, Wolfgang E., Kröner, Dietmar H., Resch, Michael M. (Eds.) (Springer, 2013).
- J. Zhou, F. Schmid, Dielectric response of nanoscopic spherical colloids in alternating electric fields: A dissipative particle dynamics simulation, J. Phys.: Cond. Matter 24, 464112 (2012).
Other references:
- S. Medina, J. Zhou, Z.-G. Wang, F. Schmid, “An efficient dissipative particle dynamics-based algorithm for simulating electrolyte solutions”, J. Chem. Phys. 142, 024103 (2015).
Scientific Contact:
Prof. Dr. Friederike Schmid
Institute of Physics
Johannes Gutenberg University Mainz
Staudingerweg 9, D-55128 Mainz (Germany)
e-mail: friederike.schmid [at] uni-mainz.de
HLRS project ID: CCAC
July 2019