MATERIALS SCIENCE AND CHEMISTRY
Topology, Entanglement and Critical Phenomena in Correlated Quantum Matter
Principal Investigator:
Fakher Assaad
Affiliation:
Lehrstuhl für Theoretische Physik I, Julius-Maximilians-Universität Würzburg
Local Project ID:
pr53ju
HPC Platform used:
SuperMUC of LRZ
Date published:
Introduction
Topology and correlations are notions that summarize one of the most active research field in the solid state. In this domain, our aim is to understand theoretical aspects of collective and emergent phenomena and their realization in nature. To achieve this goal large scale approximation free simulations are imperative, since one can only sharply define collective excitations and phase transitions in the thermodynamic limit. Clearly, this limit can never be taken, even experimentally. However, the inverse size of the system defines an energy scale, or length scale, above which one can observe such excitations and critical fluctuations. Thus, the bigger the system size, the more accurate and reliable the results. From the numerical point of view the above defines two challenges. i) developing new algorithms that are more efficient [1] and ii) optimal implementation of existing algorithms on supercomputers [2]. Below we will describe the numerical method used as well as a selection of recent results.
Results and Methods
Algorithms for lattice fermions – A general implementation of the auxiliary field Quantum Monte Carlo method.
All our projects are based on the so-called auxiliary field quantum Monte Carlo algorithm. We have been, and are, spending a lot of time and effort at developing an optimized and general OpenMP/MPI package that allows simulations of a number of models at limited programming cost. A first version of this open source project entitled the ALF (Algorithms for Lattice Fermions) can be found online and the documentation has been published in Ref. [2]. The ability to play with models of correlated electron systems at a minimal programming cost is crucial. It is a priori not clear that the models we design will deliver the collective emergent phenomena we wish to study: in many cases, simple mean-field type instabilities -- as opposed to highly entangled quantum states - will intervene. By the same token, access to computational resources that allow us to quickly and efficiently map out the nature of the phase diagram is imperative.
Correlations and frustration
Classically, frustration leads to a macroscopic degenerate ground state that violates the third law of thermodynamics. Turning on quantum effects is bound to generate new stable and potentially exotic states of matter. Typical examples of the above are quantum spin liquids, or the fractional quantum Hall effect. In Ref. [3], we have introduced a new class of coupled frustrated spin fermion models that can be simulated - free of the so-called negative sign problem - in the realm of the ALF [2]. As a case study we present in Fig. [1] results for a Kondo lattice model on the Honeycomb lattice, supplemented by frustrating couplings between the localized spins. We have shown in Ref. [3] that this coupling term generates so called partial Kondo screened states of matter, where Kondo screening becomes site dependent so as to alleviate frustration effects. To the best of our knowledge, these are the first approximation free numerical simulations that exhibit such a state of matter.

Fig.1. Inset: Kondo lattice model on the Honeycomb lattice with classical frustrating couplings between the localized spins. Main panel: Phase diagram with in-plane antiferromagnetic (xy-AFM), out-of-plane partial Kondo screening (z-PKS), spin-rotation symmetry breaking partial Kondo screening (xyz-PKS), and Kondo insulator (KI) phases from QMC simulations at T=0.025. Diamonds indicate onset of long-range order; solid (open) symbols are critical values based on L=6 and 9 (L=9 and 12) simulations. (Figure reproduced from Ref. [3]).
Competing orders in Dirac systems
In Ref. [4] we designed a model that allows for spontaneous antiferromagnetic (AFM) and Kekulé valence bond solid (KVBS) orders (See. Fig 2). We consider Dirac fermions, as realized by a tight binding model with matrix element t on the honeycomb lattice, supplemented by a Hubbard U term. This term triggers an O(3) Gross-Neveu transition between the semi-metal and an AFM. To account for a competing ordered state, we couple our model to an Ising degree of freedom in a transverse field of magnitude h. The coupling between the Ising and fermionic degrees of freedom is chosen such that when the Ising field orders it triggers a KVBS.
The main result of the paper, is the observation of a direct and continuous transition between the AFM and KVBS with emergent SO(4) symmetry. This transition cannot be understood in the realm of mean-field type Ginzburg-Landau transitions, and belongs to the category of so called deconfined quantum critical points (DQCP). Here, the emergent SO(4) symmetry allows for a topological Θ-term at Θ=π in the low energy effective field theory. As a consequence the domain wall defects of the KVBS order harbor spin ½ chains. At the critical point these spin ½ chains condense and form the AFM order.
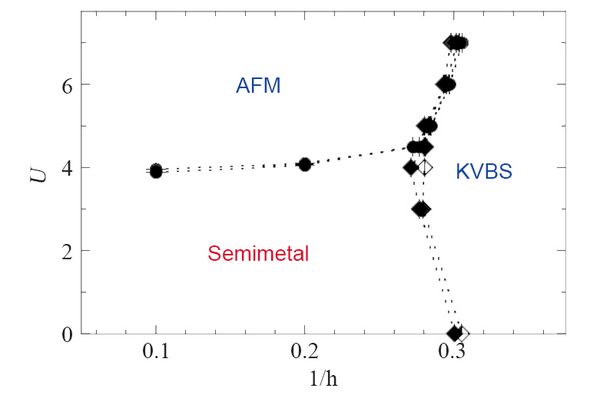
Fig. 2: Phase diagram with semimetallic, antiferromagnetic (AFM), and Kekulé-ordered (KVBS) phases from QMC simulations at T=0.05. Circles (diamonds) indicate the onset of long-range Néel (Kekulé) order; open (solid) symbols are critical values based on L=3 and 6 (L=6 and 9) simulations. (Figure reproduced from Ref. [4]).
On-going Research / Outlook
The above presents a small selection of projects, the results of which define questions and numerical challenges. In the future we will continue research along the following lines.
Algorithms. The models we have defined are free of the so called negative sign problem such that computational effort scales as the system size to the cubed times the inverse temperature. To date our Monte Carlo updating schemes are based on local updates that invariably lead to long autocorrelation times especially in the vicinity of quantum phase transitions. At present this is the major obstacle hindering access to much larger lattices. Efforts to develop global updating schemes based on machine learning or on Hybrid Monte Carlo methods are under way [5].
Competing orders in Dirac Fermions. Mutually compatible (i.e. anti-commuting) dynamically generated mass terms in Dirac systems are a golden route to study a variety of exotic quantum phase transitions where new elementary particles emerge at the transition. In the aforementioned model, we can check the validity of the low-energy effective field theory by imposing domain walls of the KVBS order and numerically testing if spin-1/2 chains emerge at the domain wall. Furthermore, a unique signature of the new so-called spinon particles, should be visible in the dynamical quantities such as the spin dynamical structure factor. Such quantities are measured in neutron scattering experiments. Finally, different mass terms, such as superconductivity and quantum spin Hall can be considered. The interest here lies in generating microscopically very different models that should belong to the same universality class.
Correlations and frustration
The PKS phase presented above, requires better understanding. In fact, the only way to define precisely Kondo physics, is with the notion of entanglement between the local spin degrees of freedom and the conduction electrons. To obtain a better understanding of the phase diagram presented in Fig. 1 we plan to study the so-called mutual information between the spin and fermion subsystems. Beyond this specific model, Ref. [3] allows for simulations of a variety of models where the spin system can be driven to an exotic state of matter such as a spin liquid. Here, notions such as Kondo breakdown where spin and electronic systems effectively decouple can be investigated.
To conclude, Quantum Monte Carlo simulations of fermion systems allow to study a variety of emergent quantum phenomena free of approximations. We can generate on the computer new phases of matter as well as new quantum phase transitions, thereby enhancing our understanding of collective quantum phenomena. Since our models are based on the fundamental rules of quantum mechanics, these states of matter should appear in nature.
References and Links
[1] Manuel Weber, Fakher F. Assaad, and Martin Hohenadler, Directed-loop quantum Monte Carlo method for retarded interactions, Phys. Rev. Lett. 119 (2017), 097401.
[2] Martin Bercx, Florian Goth, Johannes S. Hofmann, and Fakher F. Assaad, The ALF (Algorithms for Lattice Fermions) project release 1.0. Documentation for the auxiliary field quantum Monte Carlo code, SciPost Phys. 3 (2017), 013. (http://alf.physik.uni-wuerzburg.de)
[3] Toshihiro Sato, Fakher F. Assaad, and Tarun Grover, Quantum Monte Carlo simulation of frustrated Kondo lattice models, Phys. Rev. Lett. 120 (2018), 107201.
[4] Toshihiro Sato, Martin Hohenadler, and Fakher F. Assaad, Dirac fermions with competing orders: Non- landau transition with emergent symmetry, Phys. Rev. Lett. 119 (2017), 197203.
[5] Stefan Beyl, Florian Goth, and Fakher F. Assaad, Revisiting the hybrid quantum Monte Carlo method for Hubbard and electron-phonon models, Phys. Rev. B 97 (2018), 085144.
[6] https://alf.physik.uni-wuerzburg.de
Researchers
F.F. Assaad(PI), F. Goth, J. Hofmann, F. Parisen Toldin, T. Sato, Z. Wang (all: University of Wuerzburg, Department of Theoretical Physics I)
Scientific Contact
Prof. Dr. Fakher Assaad
Theoretische Physik I
Julius-Maximilians-Universität Würzburg
Am Hubland, D-97074 Würzburg (Germany)
e-mail: fakher.assaad [@] physik.uni-wuerzburg.de
NOTE: This report was first published in the book "High Performance Computing in Science and Engineering – Garching/Munich 2018".
Local project ID: pr53ju
December 2019