ENGINEERING AND CFD
Dynamics of Multi-Component Fluid Dynamics in Porous Structures
Principal Investigator:
Mauro Sbragaglia
Affiliation:
University of Roma (Italy)
Local Project ID:
PP13081531
HPC Platform used:
Hermit of HLRS
Date published:
The dynamics of multi-component and/or multi-phase flows in porous media is crucial for many disciplines and many applications as, e.g., for oil recovery techniques enhancement, the dispersion of pollutants in aquifers, filtration and centrifuging in many chemical engineering applications and wetting of feathers, the air dynamics in lungs and the design of bone tissue perfusion microreactors [1]. In this project, scientists aimed to perform a systematic investigation of multi-component and/or multi-phase flows dynamics in porous matrices adopting a bottom-up, multi-scale approach, based on a Lattice Boltzmann Method (LBM) [2].
LBM is an optimal form of Boltzmann kinetic equation describing the dynamics of a fictitious ensemble of particles, whose motion and interactions are confined to a regular space-time lattice. The huge separation of scales, needed to resolve the fluid-fluid and the fluid-solid interfaces, together with the porous matrix structures asks for massive parallel computations on Tier-0 systems. In collaboration with Prof. F. Toschi at TU/e (University of Eindhoven, The Netherlands) and the PhD student Mr. R. Scatamacchia (University of Rome, Italy), project leader Mauro Sbragaglia of the University of Rome performed simulations based on three different configurations: (a) a standard T-junction, where a dispersed phase is injected perpendicularly into the main channel containing a continuous phase; (b) a modified Y-Junction obtained by allowing a change in the angle which the side channel forms in connection with the main channel (see figure 1); (c) a multi-component simulation in a porous media using different wetting boundary conditions and different pore-size distribution, both homogeneous and heterogeneous.

Figure 1 Dynamics and Break-up of droplets in T/Y-junctions from LBM simulations. Various junctions can be realized with a specific numerical approach by allowing the side channel to meet the main channel with various angles: θ = 0◦ (Panels (a)-(c)); θ = 25◦ (Panels (d)-(f )); θ = 45◦ (Panels (g)-(i)). The dispersed phase enters the main channel and periodically breaks due to the forces created by the cross-flowing continuous phase. The various snapshots are equi-spaced in time. The emulsion time (a constant for the simulations) as unit of time was chosen.
Copyright: Department of Physics and INFN, University of Rome 'Tor Vergata'In both cases (a)-(b) the scientists investigated the dynamics and break-up processes of Newtonian droplets. They characterized the conditions for the existence of break-up mechanisms and study the critical range of Capillary numbers at which the system transits from a squeezing mechanism into a shear-dominated droplet break-up, at changing the junction geometry (see figure 1). Preliminary data analysis shows that the effect of the geometrical change enhances a dripping mechanism, promoting smaller droplet size with enhanced frequency of formation.
The researchers also performed multi-component simulations in a porous media using different wetting boundary conditions and different pore-size distribution in a plain closed channel (see figures 2 and 3). Preliminary data analysis suggest very promising results. The scientists are going to be able to assess the statistics of the velocity components both along and transverse to the imposed flow direction, even with residual trapping of a second immiscible fluid. Moreover, pore-scale correlations in the flow are found that are determined by the geometry of the medium.

Figure 2: Immiscible fluids displacement in heterogeneous porous media out of numerical simulations based on the Lattice. Boltzmann Models (LBM).
Copyright: Department of Physics and INFN, University of Rome 'Tor Vergata'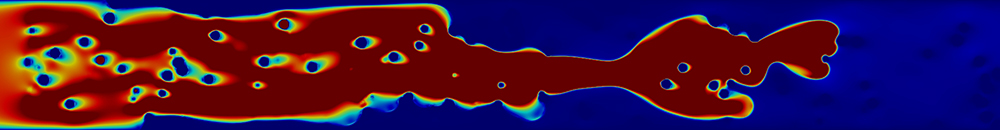
Figure 3: Magnitude of the velocity field in the displacing fluid in heterogeneous porous media (see figure 2). Low velocity regions appear dark (blue) while high velocity regions are light (red).
Copyright: Department of Physics and INFN, University of Rome 'Tor Vergata'This project was made possible through the Partnership for Advanced Computing in Europe (PRACE) using the HPC infrastructure of HLRS Stuttgart.
[1] J. Bear, Dynamics of fluids in porous media, Elsevier (1972)
[2] S. Succi, The lattice Boltzmann Equation, Oxford Science (2001)
Mauro Sbragaglia
Associate Professor in Theoretical Physics, Mathematical Models and Methods
Department of Physics and INFN, University of Rome 'Tor Vergata'
Via Della Ricerca Scientifica 1, I-00133 Rome/Italy
http://people.roma2.infn.it/~sbragaglia/