ENVIRONMENT AND ENERGY
Model Development for Meteorological Applications
Principal Investigator:
Martin Baumann
Affiliation:
Universitätsrechenzentrum, Ruprecht-Karls-Universität Heidelberg (Germany)
Local Project ID:
hka14
HPC Platform used:
JUQUEEN of JSC
Date published:
The dynamic behavior of the atmosphere is driven by processes on a wide range of spatial and temporal scales. In a project run by scientists of the Heidelberg University, those parts of model systems which describe the fluid dynamics and the temperature evolution were investigated. The models are formulated in terms of the velocity, temperature, pressure, and density. The researchers employ a hierarchy of different physical models with an increasing degree of complexity. The task of predicting the evolution of tropical cyclones is a typical challenging example.
Part 1: Numerical Experiments using a Decoupled Solution Scheme
A scheme for the solution of incompressible Navier-Stokes equations with Boussinesq approximation was studied which decouples the fluid dynamics and the temperature evolution. The scheme is composed of two solvers which run on separate sets of processors. This allows to adjust the parallel configuration to the individual solver needs and thus improve efficiency. After each time step, the solvers update each other with the new state. The communication is done with the OpenPALM tool which arranges the parallel transfer of the distributed data between the individual processors of both solvers.
Part 2: Comparison of Compressible Fluid Flow Models for a Cyclone-Cyclone-Interaction Scenario.

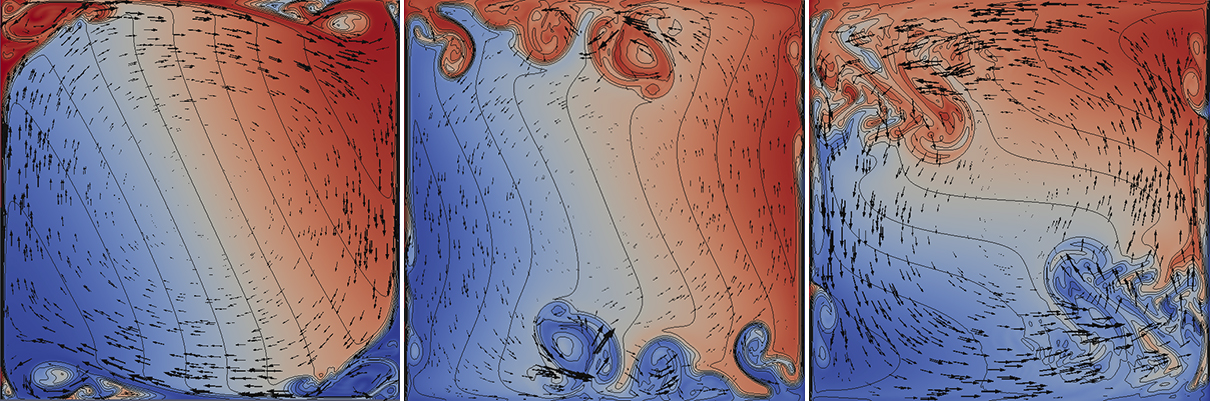
Illustration 1: Scenario of interacting idealized tropical cyclones: (a) Isosurfaces of the vorticity and streamlines of the velocity field for the result of an incompressible Navier-Stokes model. (b) Isosurfaces of the vorticity and glyphs of the velocity field for the result of a compressible Navier-Stokes model.
Copyright: URZ, Ruprecht-Karls-Universität Heidelberg, GermanyThe accurate prediction of the motion and evolution of tropical cyclones (TCs) is a challenging problem for numerical weather prediction, because interacting physical processes on a wide range of scales have to be considered. In this subproject, a scenario of two idealized tropical cyclones was used as a benchmark problem for the analysis of the simulation results that can be determined using different physical models. While a strongly idealized 2D model and a 3D incompressible version of the Navier-Stokes Equations was considered in previous works, two more complex models where in the focus of this investigation: The compressible Navier-Stokes equations as well as the Low-mach number approximation were considered for the simulation of a cyclone-cyclone-interaction scenario. Discrete versions of the two models were implemented in one unified framework using the open-source software package HiFlow3. For the discretization in space, bi-linear finite elements (Q1-elements) with streamline upwind Petrov-Galerkin (SUPG) stabilization for the convective terms and PSPG/div-div (Pressure-Stabilizing/Petrov–Galerkin) stabilization for the Low-mach model was used. Both models feature a Crank-Nicolson discretization in time.
Part 3: Reference Scenario "Baroclinic Wavetank"
In contrast to laboratory experimental set-ups, measurements of an atmospheric state can be done only once and the identical state can never be reproduced again. Therefore, it is difficult to determine reference experiments based on which numerical models of systems can be validated adequately. A partially heated, rotating annulus was considered: The baroclinic wavetank scenario. Using this laboratory experiment, waves can be created that can be interpreted as idealized baroclinic waves in the atmosphere.
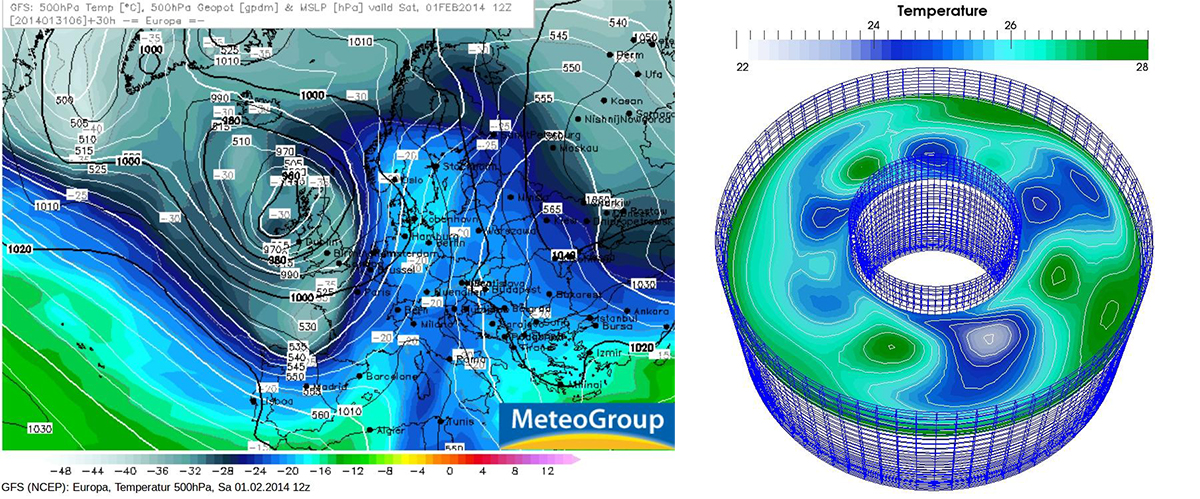
This scenario has been chosen within the DFG SPP MetStröm (1276) as a reference experiment based on which software packages and discretization and solutions procedures can be benchmarked. The numerical simulations for this investigation where conducted using HiFlow3. The incompressible Navier-Stokes equations with a Boussinesq approximation for the buoyancy force were considered and a stable finite element method (Q2/Q1/Q2) for (vel./pres./temp.) was used in space. The time-discretization was done using the Crank-Nicolson scheme with a time step size of 0.25 sec for physical simulation times of 30 minutes per experiment. In each time-step a fully coupled nonlinear problem was solved using Newton's method resulting in several thousand linear systems to be solved, each with about 2-16 million unknowns.
Research Team:
Martin Baumann, Simon Gawlok, Martin Wlotzka, Philipp Gerstner, Teresa Beck, Vincent Heuveline
Engineering Mathematics and Computing Lab (EMCL)
Interdisciplinary Center for Scientific Computing (IWR)
Heidelberg University, Germany
Scientific Contact:
Dr. Martin Baumann
Servicebereich Future IT - Research & Education (FIRE)
Ruprecht-Karls-Universität Heidelberg, Universitätsrechenzentrum (URZ)
Im Neuenheimer Feld 293, D-69120 Heidelberg (Germany)
e-mail: martin.baumann [at] urz.uni-heidelberg.de
http://www.urz.uni-heidelberg.de