ASTROPHYSICS
The Sonic Scale Revealed by the World’s Largest Turbulence Simulation
Principal Investigator:
Christoph Federrath (1), Ralf S. Klessen (2)
Affiliation:
(1) Research School of Astronomy and Astrophysics, Australian National University (ANU), (2) Zentrum für Astronomie, Institut für Theoretische Astrophysik und Interdisziplinäres Zentrum für Wissenschaftliches Rechnen, Universität Heidelberg (Germany)
Local Project ID:
pr32lo
HPC Platform used:
SuperMUC of LRZ
Date published:
Introduction
Understanding turbulence is critical for a wide range of terrestrial and astrophysical applications. For example, turbulence on earth is responsible for the transport of pollutants in the atmosphere and determines the movement of weather patterns. But turbulence plays a central role in astrophysics as well. For instance, the turbulent motions of gas and dust particles in protostellar disks enables the formation of planets. Moreover, virtually all modern theories of star formation rest on the statistics of turbulence (Padoan et al., 2014). The theoretical assumptions about turbulence behind star formation theories allow the prediction of star formation rates in the Milky Way and in distant galaxies (Salim et al., 2015; Sharda et al., 2018). Interstellar turbulence shapes the structure of molecular clouds (Klessen & Glover, 2016) and is a key process in the formation of filaments, the building blocks of star-forming clouds.
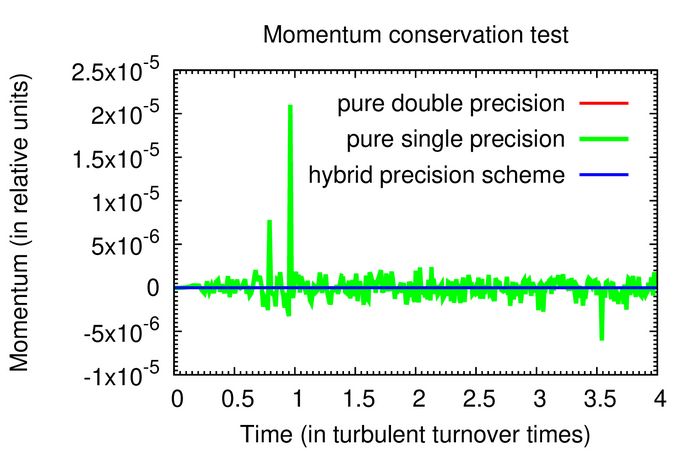
Figure 1: Comparison of the pure double-precision and pure-single precision schemes with our new hybrid-precision scheme for modelling su- personic and subsonic turbulence. The mass and momentum are well conserved in our hybrid-precision scheme, shown as a straight blue line (identical to the pure double-precision scheme), while significant errors arise in the pure single-precision scheme (shown as the green line).
Copyright: ANUA key ingredient for all these models is the so-called sonic scale. The sonic scale marks the transition from supersonic to subsonic turbulence and produces a break in the turbulence power spectrum from E ∝ k−2 to E ∝ k−5/3, or equivalently in the 2nd-order velocity structure function from SF2 ∝ l1/2 (in the supersonic regime) to SF2 ∝ l1/3 (in the subsonic regime). While these structure function slopes of 1/2 and 1/3 for the supersonic and subsonic parts of the spectrum have been measured independently, there is no simulation currently capable of bridging the gap between both regimes. This is because previous simulations did not have enough resolution to separate the injection scale, the sonic scale and the dissipation scale.
The aim of this project is to run the first simulation that is sufficiently resolved to measure the exact position of the sonic scale and the transition region from supersonic to subsonic turbulence. We therefore ran a simulation with the unprecedented resolution of 10,0483 grid cells on SuperMUC, in order to resolve the sonic scale.
Results
In the framework of a GCS Large-Scale Project, an allocation exceeding 40 million core hours has been granted to this project on LRZ HPC system SuperMUC. The simulation code used for this project is FLASH, a public, modular grid-based hydrodynamical code for the simulation of astrophysical flows (Fryxell et al., 2000). The parallelisation is based entirely on MPI. In the framework of the SuperMUC Phase 2 scale-out workshop, the current code version (FLASH4) has been optimised to reduce the memory and MPI communication requirements. In particular, non-critical operations are now performed in single precision, without causing any significant impact on the accuracy of the results (see Figure 1). In this way, the code runs with a factor of 4.1 less memory and 3.6 times faster than the version used for the previous large-scale project at LRZ (Federrath, 2013), and scales remarkably well up to the full machine on SuperMUC Phase 2 (Hammer et al., 2016).
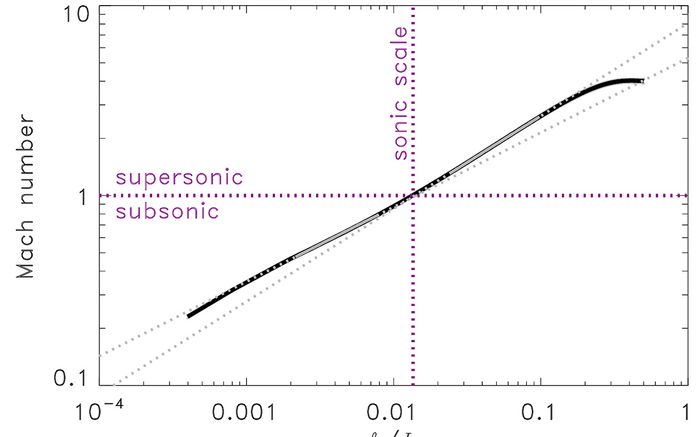
Figure 2: Velocity structure function of the simulation with 10,0483 cells. This reveals the transition from supersonic to subsonic turbulence around the sonic scale (defined as the scale where the Mach number is unity). We find the sonic scale at about 1/100th of the computational box size.
Copyright: ANUOur current 10,0483 simulation has been completed and data processing is in progress. The simulation was run on 65,536 com- pute cores, used up the full allocation of 40 million core hours and produced about 2 PB of output data. Here we present the first results of the simulations, with a focus on identifying the sonic scale.
In order to find the sonic scale we computed the 2nd-order velocity structure functions over a period of 5 large-scale turbulent turnover times. Figure 2 shows the time-averaged structure function (with error bars quantifying fluctuations in time around the average), where we have plotted the Mach number (defined as √SF2 /cs , where cs is the isothermal sound speed of the gas), as a function of scale l/L (in units of the size of the computational domain, L). We can directly use this plot to identify the position of the sonic scale and transition region around it. We find the sonic scale where the Mach number is unity, which gives a sonic scale of l/L ∼0.014. Power-law fits in the subsonic and supersonic regime yield slopes of 0.4 and 0.5, respectively, close to the theoretical expectations (the subsonic slope is slightly steeper than the original Kolmogorov prediction of 1/3, likely because of necessary intermittency corrections; see Schmidt et al., 2008). The transition region around the sonic scale is about a factor of 3 in l.
We can use the measured position and width of the sonic scale from Figure 2 to visualise the density structures associated with the sonic-scale transition. We do so in Figure 3, which shows the gas density in the entire domain (left-hand panel) and the Fourier-filtered density field to highlight the density structures around the sonic scale (scales of about 1/100th of the box size; shown in the right-hand panel). This reveals the position and morphology of the sonic-scale structures. We find that they are associated with strong shocks, i.e., the transition regions between pre-shock and post-shock gas. The filaments and sheets tracing these structures have enormous density contrasts of 100–1000.
These sonic-scale structures are key ingredients for star formation. We think that they are associated with the formation of interstellar filaments (Federrath, 2016). Dense cores may form at the intersection of such filaments, which marks the onset of local gravitational dominance of these cores, such that they can proceed via gravitational collapse to form stars. Hence, the sonic scale is a key ingredient in star-formation theory (Krumholz & McKee, 2005; Federrath & Klessen, 2012).
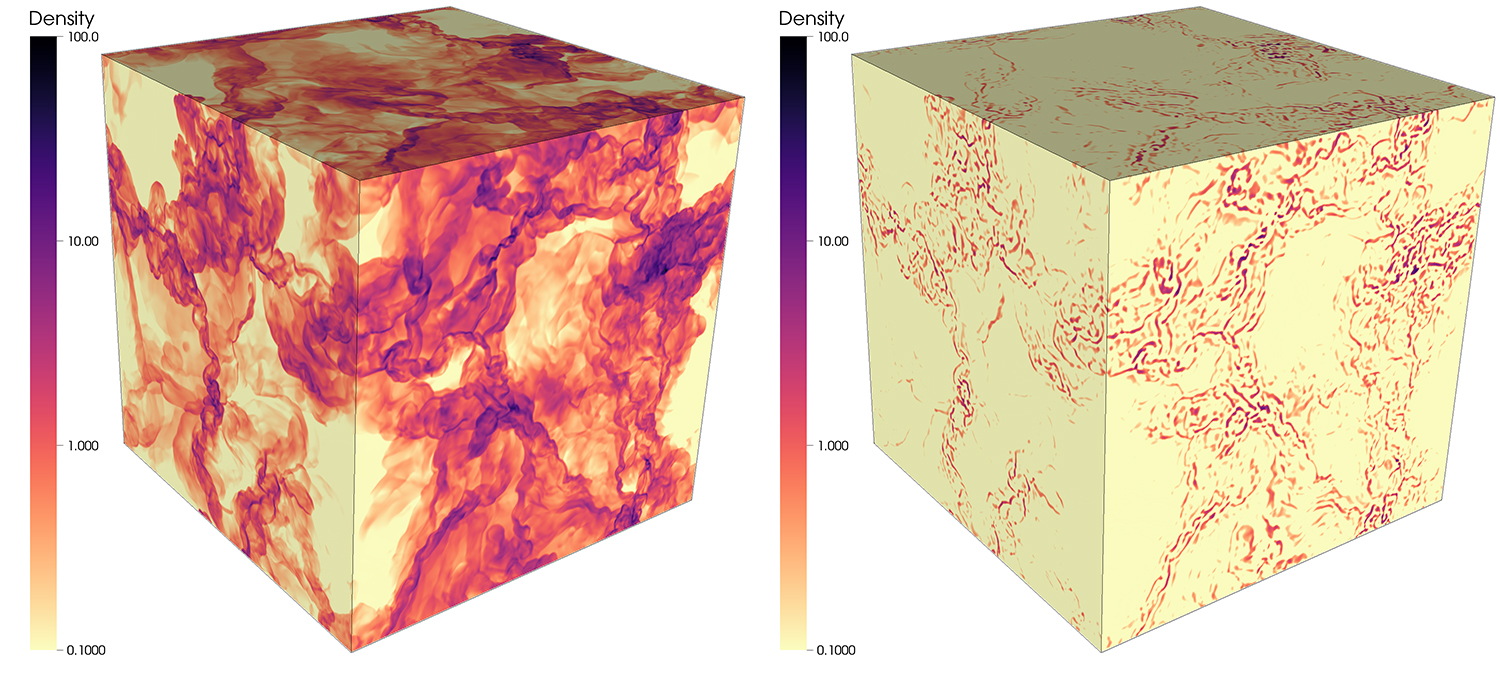
Figure 3: Three-dimensional visualisation of our turbulence simulation. The left-hand panel shows the gas density in the entire domain, while the right-hand panel highlights those structures that are associated with the sonic scale. We see that these sonic-scale structures trace the edges of strong shocks. The density contrasts are up to 1000 across these sonic surfaces.
Copyright: ANUThe visualisation shown in Figure 3 highlights the enormous complexity of the turbulent structures on all spatial scales covered in these simulations. For further visualisations and movies of the simulation, please visit http://www.mso.anu.edu.au/~chfeder/pubs/extreme_scaling/extreme_scaling.html
There are many other fundamental aspects of turbulent flows that can be studies with this large simulation (fractal dimension, probability distribution functions of key dynamic variables, etc.).
This is work in progress.
Researchers
Christoph Federrath1, Ralf S. Klessen2,3, Luigi Iapichino4
1 Research School of Astronomy and Astrophysics, Australian National University (christoph.federrath [@] anu.edu.au)
2 Universität Heidelberg, Zentrum für Astronomie, Institut für Theoretische Astrophysik (klessen [@] uni-heidelberg.de)
3 Universität Heidelberg, Interdisziplinäres Zentrum für Wissenschaftliches Rechnen
4 Leibniz-Rechenzentrum der Bayerischen Akademie der Wissenschaften (luigi.iapichino [@] lrz.de)
References and Links
Federrath, C. 2013, Monthly Notices of the Royal Astronomical Society, 436, 1245
Federrath, C. 2016, Monthly Notices of the Royal Astronomical Society, 457, 375
Federrath, C., & Klessen, R. S. 2012, The Astrophysical Journal, 761, 156
Fryxell, B., Olson, K., Ricker, P., et al. 2000, The Astrophysical Journal Supplement Series, 131, 273
Hammer, N., Jamitzky, F., Satzger, H., et al. 2016, Advances in Parallel Computing, 27, 827
Klessen, R. S., & Glover, S. C. O. 2016, Star Formation in Galaxy Evolution: Connecting Numerical Models to Reality, Saas-Fee Advanced Course, 43, 85
Krumholz, M. R., & McKee, C. F. 2005, The Astrophysical Jour- nal, 630, 250
Padoan, P., Federrath, C., Chabrier, G., et al. 2014, Protostars and Planets VI, 77
Salim, D. M., Federrath, C., & Kewley, L. J. 2015, The Astrophys- ical Journal Letters, 806, L36
Schmidt, W., Federrath, C., & Klessen, R. 2008, Physical Review Letters, 101, 194505
Sharda, P., Federrath, C., da Cunha, E., Swinbank, A. M., & Dye, S. 2018, Monthly Notices of the Royal Astronomical Society
Scientific Contact:
Dr. Christoph Federrath
Research School of Astronomy and Astrophysics
The Australian National University
Cotter Road, Canberra, ACT 2611, Australia
Email: christoph.federrath[at]anu.edu.au
http://www.mso.anu.edu.au/~chfeder
Uni Heidelberg: http://www.ita.uni-heidelberg.de/~chfeder/pubs/supersonic/supersonic.shtml?lang=en
NOTE: This report was first published in the book "High Performance Computing in Science and Engineering – Garching/Munich 2018".
LRZ project ID: pr32lo
March 2019